Source Modeling and Astrophysics

Relating extreme astrophysical events to their gravitational-wave emission in an accurate and efficient manner is the main focus of Source Modeling. Numerical relativity and analytical relativity meet to inform waveform models that experimentalists have used to detect and characterize gravitational waves from the collision of black holes and neutron stars during the observing runs of Advanced LIGO and Virgo.

Why source modeling?

Fig. 1: Lobes of gravitational radiation projected onto the orbital plane of a nonspinning, unequal-mass black-hole binary.

In extreme astrophysical events where large masses move at speeds close to the speed of light, characteristic gravitational-wave signals are generated. When on the lookout for gravitational waves via interferometric experiments, data analysts have to battle against lots of different sources of noise (environmental, instrumental, thermal, …) that may hide the sought-after signals. Ideally, one would like to know what to look for in the data as to tell noise and gravitational waves apart. This means predicting what are the plausible gravitational waveforms of astrophysical origin in the frequency range that is accessible to the experimental setup. For frequencies between about 10 and 1000 Hz (the current state-of-the-art for ground-based interferometers), the loudest sources of gravitational waves are coalescing binaries of compact objects: in fact, the LIGO/Virgo/Kagra Scientific Collaboration is routinely detecting black hole mergers and neutron star mergers, and uses these detections to learn about the fundamental nature of gravity, and about the astrophysical black holes and neutron stars in our Universe. These compact object binaries are the main focus of our Department. The motivation for this line of research is also theoretical, concerning the problem of solving the equations of General Relativity in such challenging scenarios. In a few words, the goal of source modeling is to relate the astrophysics of the sources of gravitational waves to the observations in the most efficient way.

What is the gravitational-wave signature of compact-object binaries?

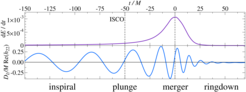
Under General Relativity, two objects orbiting each other emit gravitational radiation, and undergo four stages: their orbit shrinks in the inspiral stage, resulting in a plunge stage and final coalescence (merger stage). Thereafter they settle to a final remnant (ringdown stage). For the highly dynamical regime close to merger, numerical simulations must be used to obtain an accurate description of the system for the construction of templates for detectors. This is vital for detectors to achieve their detection potential and to connect gravitational-wave data to the properties of black hole systems.

Fig. 2: Stages of black-hole binary coalescence: (from left to right) inspiral, plunge, merger, and ringdown.
Fig. 3: The energy flux in gravitational waves and the dominant quadrupolar mode of gravitational radiation. ISCO refers to the innermost stable circular orbit. Bodies whose separation is smaller must plunge towards each other.


 

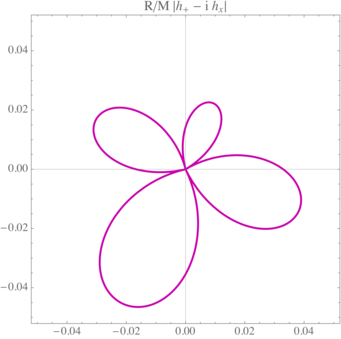
A lot of the effort in the department is geared towards numerical modeling of black hole binaries. These simulations are computationally intensive and currently are feasible only for a limited number of binary configurations out of the whole 7-dimensional parameter space, parametrized by mass ratio and black hole angular momenta (or spin). One particular area of focus is the exploration of systems in which the misalignment of the black-hole spins with the orbital angular momentum causes the orbital plane and the spins to precess. This precession is imprinted on the gravitational waveform and a detailed understanding of its effects is important to extract the maximum amount of information about gravitational-wave sources.
Another major area is expanding the boundaries of computational ability to simulate high mass ratio (> 8), high spin (up to dimensionless spin of 0.9) binaries with tens of orbits. Such systems are predicted to be of astrophysical interest and can serve as proxies for neutron star - black hole binaries.

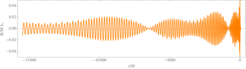
Fig. 4: Waveform emitted by a precessing black hole binary displaying strong amplitude modulations.

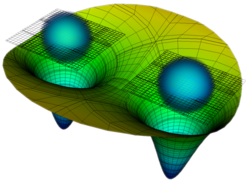
Fig. 5: Computational setup used for binary neutron star evolutions.

Members of our department have also been investigating the coalescence of binary neutron stars, which are central engines for short gamma ray bursts and kilonovae and one source for heavy elements present in our Universe. These violent events are sources of both gravitational wave emission and electromagnetic radiation. Inside neutron star stars matter exists at pressures and densities that are out of reach in terrestrial laboratories. Therefore, neutron stars and in particular neutron star collisions allow to constrain the unknown cold supranuclear equation of state. Simulating binary neutron stars presents an extra challenge due to the complexity of the equations describing the matter fields. However, we have succeeded in simulating long and accurate gravitational waveforms lasting tens of gravitational wave cycles in a large region of the binary neutron star parameter space performing the first precessing binary neutron star simulations as well as studying systems with the highest mass ratio simulated so far. The obtained knowledge has been directly used to interpret the first binary neutron star observation.

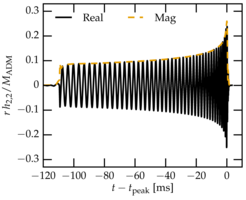
Fig. 6. The dominant quadrupolar mode of gravitational radiation, showing the inspiral and merger of 2 neutron stars and subsequent formation and ringdown of a black hole.

Solving Einstein's equations numerically requires one to recast them in a form suitable for time evolution. Since the Numerical Relativity breakthrough in 2005 many approaches have been developed. Our department uses mostly the Spectral Einstein Code (SpEC) developed jointly by many institutions. SpEC uses pseudo-spectral methods to achieve very high accuracy with very modest resources used. High fidelity simulations are essential for using numerical relativity in constructing gravitational wave templates, as interferometric detectors are very sensitive to phase error that accumulates throughout the coalescence. Due to the high accuracy of spectral methods, SpEC can be used to perform very long numerical relativity simulations that can last up to 100 gravitational wave cycles, facilitating the creation of very long templates needed by advanced era detectors and the calibration and study of semi-analytical approximations.

Fig. 7: Diagram summarizing the main idea behind the effective-one-body model for the dynamics of black hole binaries.

Members of our department have also expertise and participated in the development of other numerical codes (most notably BAM and the Einstein Toolkit). These codes have also been very successful in investigating mergers of compact object binaries. Both codes use finite-differences to discretize the space-time which offers flexibility and robustness when applied to a wide variety of systems and complements the investigations done with SpEC.

How does our Department address the needs of data analysts?

The number of possible binary configurations is very large, so that a purely numerical-relativistic approach is neither feasible nor practical. To make things even more challenging, one needs waveform generation times that are orders of magnitude smaller than what is currently possible for numerical-relativity codes.

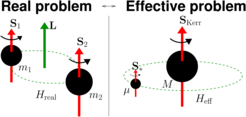
Our Department works on building semi-analytical models that are inexpensive to evaluate but can still provide accurate predictions of the waveforms. A very successful approach, that goes under the name of Effective One Body model, starts from perturbative expansions of General Relativity, i.e. the post-Newtonian and the black-hole perturbation theory frameworks, respectively, and aims at bridging their respective domains of validity via a unified formalism. In this model, one maps the dynamics of a binary black hole to the motion of a test mass in an effective background spacetime. The effective spacetime is a deformation of the Kerr solution that describes a spinning black hole. The deformation is proportional to the mass ratio of the binary in the real problem. The dissipative dynamics is obtained from appropriate resummations of post-Newtonian formulae for the gravitational waveforms. This model relies on a set of ordinary differential equations that are fast to solve, as compared to the full fledged general relativistic theory, and it can be naturally tuned to numerical-relativity simulations to improve its predictions.

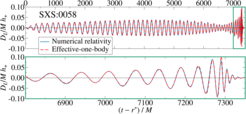
Fig. 8: Comparison of the effective-one-body model to a numerical-relativity waveform of a precessing black-hole binary.


The model has been validated through hundreds of comparisons to numerical relativity, concerning both the conservative dynamics and the radiation. A highlight is the construction of a highly accurate model for black-hole binaries with generic spins that can generically undergo precession. This phenomenon is responsible for extremely complicated gravitational-wave signals, but the great complication is balanced by the wealth of information about the source that the waveform encodes. Thanks to the prominent role we play in the LIGO collaboration, the models that we develop are a core tool for LIGO data analysis. In particular, the nonprecessing, black-hole binary model developed by members of our Department was used by the template-based detection pipelines that assessed the significance of the first gravitational-wave observations during the first observing run of Advanced LIGO. Also, our models (both spin-aligned and spin-precessing) were used for parameter-estimation studies of recent events. Our models will be actively used by the LIGO Compact Binary Coalescence detection and parameter-estimation pipelines during the next observing runs of Advanced LIGO and Virgo.

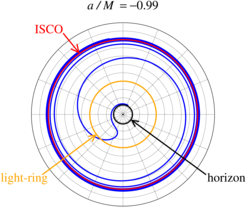
Fig. 9: Trajectory of a point mass plunging into a Kerr black hole that is spinning.

In collaboration with researchers at other institutions, we are also actively exploiting black-hole perturbation theory to investigate the merger of black-hole binaries with extreme mass ratios, that is when one of the two black holes is significantly more massive than the companion. The main goal of this line of research is the extraction of strong-field information about the plunge of particles into spinning black holes. Such information can be folded into effective-one-body models of comparable-mass black-hole binaries to enforce their correct extreme-mass-ratio limit. Fully relativistic phenomena (like quasinormal mode mixing) can be explored with ease for a large number of configurations by numerical solution of perturbation equations.

When one of the two compact object in a binary is a neutron star, a whole new range of possible physical processes opens up, thanks to the presence of highly dense matter in the source. This results in a rich phenomenology of waveforms that allow us to probe the properties of neutron-star matter. Members in our department have extended our black-hole binary models to the cases including neutron stars. Tidal and resonant effects of neutron stars are being explored and compared to what is seen in numerical-relativity simulations.

Astrophysical aspects of source modeling

From the astrophysical point of view, we study gravitational wave sources from all sizes, from stellar masses to the supermassive ones. Stellar-mass binaries are detectable with the Advanced ground-based detectors LIGO/Virgo, and we use relativistic stellar dynamics to study their properties and event rates. If clusters harbour intermediate mass black holes (with masses between a hundred times the mass of the Sun up to a hundred thousand times that mass) in their centres, they can become a strong source of gravitational waves when they merge, and can be observed with aLIGO/Virgo and LISA in a matter of a few tens of days. This a very exciting possibility in terms of synergy between the two observatories, and this has been a point we have addressed in the last few years. A small stellar-mass black hole inspiraling towards a supermassive one is one of the most interesting sources we expect to detect with the proposed Laser Interferometer Space Antenna (LISA). They have the potential to reveal the astrophysics of galaxy centers and their black holes, as well as to test general relativity in the strong field regime. We are also studying the fate of the black hole binaries formed during galaxy mergers, in particular the question whether the black holes merge or stall at sub-parsec separations. We focus on the evolution in gas-rich environments, from parsec separations until they reach the gravitational-wave-driven phase.

Questions addressed by source modeling and astrophysics:

  • How do gravitational waves emitted by astrophysical sources look like?
  • How do the waveforms depend on the presence of dense matter in the source?
  • How can we predict waveforms in the fastest possible way?
  • How do the waveforms depend on the intrinsic parameters of the source?
  • What is the mapping between galaxy mergers and mergers of their host massive black holes?
  • What are the dynamical mechanisms and their relative weights that affect EMRIs? What are the physical conditions around EMRIs?
  • In terms of IMRI detections, what is the most valuable scientific return from the perspective of astrophysicists and cosmologists, as regards of mass function of SMBHs, IMBHs, and cosmological evolution?

Web-View
Print Page
Open in new window
Estimated DIN-A4 page-width
Go to Editor View